Q1 - A particle's position is defined by x - 6t^3 - 22t^2, where x is expressed in meters and t in seconds. Determine (a) the times when the velocity in zero, (b) the million when the acceleration is zero.
Q2 - Acceleration of a particle in defined by a -90y^(-2.5), where y is m and a is :m/s^2. If starting at rest at y = 3 m, determine the velocity when (a) y = 2 m and y = 2 mm.
Q3 - A particle enters a fluid at x =0 with a velocity of 520 fps and moves 10 inches before coming to rest. Assume velocity v = vo - kx where v and x are fps and ft. Determine the (a) initial acceleration and (b) time to penetrate 9.7 inches into the fluid.
Q4 - A local elevator moves upward at a constant 4.5 mps passing a stopped express elevator. Five seconds later the express elevator starts upward with a constant acceleration of 2.4 mpsps. Determine (a) the speed of the express elevator and (h) how high it climbed when it reached the local elevator and (c) how long it took.
Q5 - A cannon ball is fired from the 5 m high tower shown with a velocity of 28 m/s and an angle theta = 70 deg. Determine the (a) flight time in s, (b) coordinate location of ground strike and (c) speed of the ground strike if the slope has a rise/run = 2/5 and the horizontal dimension dis = 9m.
Q6 - A particle moves along the path y -0.02 x^4 - 2 (meters) with a constant speed in the Cartesian X-component of velocity vx = + 4 m/s. Determine (a) the Cartesian Y-component of Velocity and the (b) normal and (c) tangential components of acceleration when the particle is at x = -2 m.
Q7 - A block moves outward along the slot in the platform with a speed of dr/dt = 4t^4 m/s where t is in seconds. The platform rotates at a constant rate of omega = 2.5 rad/s. If the block starts from rest at r = 0 (the center), determine the magnitudes of (a) its velocity and (b) acceleration when the block is at position = 11 m.
Q8 - In pulley system shown, the velocity of block C with respect to block A is 20 m/s. Determine (a) the velocity of block Li and (b) the velocity of block A with respect to block B.
Q9 - Sliders A and B are connected to the cord that pusses over a pulley of negligible size located at AA = 8 and BB = 8 as shown. At the instant shown, the positions of sliders A and B are SA = 21 ft and SB = 9 ft and slider. A is moving upward at a constant velocity of 9 fps.
Determine the magnitudes of (a) the velocity and (b) acceleration of slider B with respect to slider A.
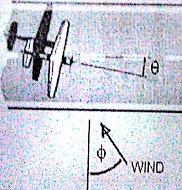
Q10 - An airplane's airspeed (speed relative to still air) is 105 km/h. The wind speed relative to the ground is 44 km/h and is directed at phi = 65 degrees as shown. Determine (a) the angle theta at which the plane must be directed (steered) in order to travel in the direction of the runway. Also (b) determine the speed of the airplane relative to the runway.
Q11 - A camera rotates on a tripod while tracking the motion of a particle traveling the path y = 12 -11x -3x^2 where x and y are coordinate locations in m. At the instant shown, the particle's x-coordinate location is x = -4 m with a speed of 45 m/s that is increasing at a rate of 50% second.
If the camera is positioned at the origin shown, determine camera's (a) the angular velocity and the angular acceleration.
